weightlossvasup.blogg.se - Alternating harmonic series

#ALTERNATING HARMONIC SERIES HOW TO#
#ALTERNATING HARMONIC SERIES SERIES#

Find N Arithmetic Means between A and B.

Find Harmonic mean using Arithmetic mean and Geometric mean.

#ALTERNATING HARMONIC SERIES SERIES#

This is an alternating geometric series with. We will refer to the factor as the alternating symbol. A typical alternating series has the form where for all.

Program to find the Nth Harmonic Number An alternating series is an infinite series whose terms alternate signs.

ISRO CS Syllabus for Scientist/Engineer Exam.

ISRO CS Original Papers and Official Keys.

GATE CS Original Papers and Official Keys this exercise we have to find to Siris ultimate it Siris such that the product of the tools um um Isam let the product off each term isa the emergencies. Letters to the Editor The M a t h e m a t i c a l lntelligencer The Alternating Harmonic Series I e n j o y e d Friesecke and Wehrstedt's Note, 'An Elementary Proof of the Gregory- encourages c o m m e n t s a b o u t the Mengoli-Mercator Formula' (Mathematical hltelligeJ:lcer 28 (2006), no.

This result is known as the Riemann Rearrangement Theorem, which is beyond the scope of this book. The notion that alternating the signs of the terms in a series can make a series converge leads us to the following definitions.

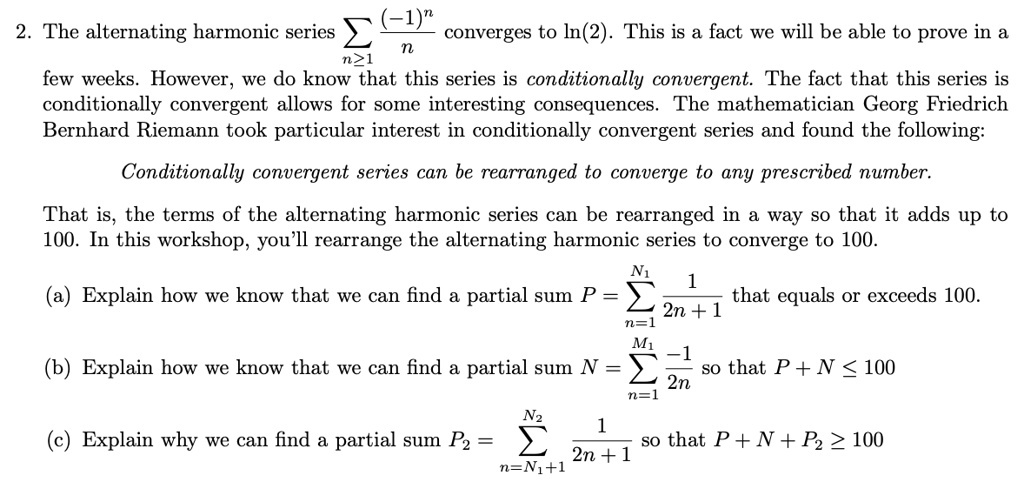
For any series that converges absolutely, the value of is the same for any rearrangement of the terms. One of the famous results of mathematics is that the Harmonic Series, n 1 1 n diverges, yet the Alternating Harmonic Series, n 1 (-1) n + 1 1 n, converges. A series that converges absolutely does not have this property. In general, any series that converges conditionally can be rearranged so that the new series diverges or converges to a different real number. EX 1 Does an Alternating Harmonic Series converge.

#ALTERNATING HARMONIC SERIES HOW TO#

In (Figure), we show how to rearrange the terms to create a new series that converges to We point out that the alternating harmonic series can be rearranged to create a series that converges to any real number however, the proof of that fact is beyond the scope of this text. The error made by estimating the sum, Sn is less than or equal to an+1, i.e. The terms in the alternating harmonic series can also be rearranged so that the new series converges to a different value. Slightly less well known is that the alternating harmonic series converges to a very clean limit - strangely enough, it turns out to be ln 2. It converges by the alternating series test, but the sum of its absolute values is the harmonic series, which diverges. As a byproduct of this proof (and the associated technique) we arrive at the conv ergence of the. It is well known that the harmonic series diverges. the alternating harmonic series could be termed the more harmonious sibling of the harmonic series. Combinatorial series identities, Summation formulas, Partial fraction approach, Alternating harmonic numbers, Binomial coe cients, Integral representation. which, of course, is just the harmonic series with the sign of every other term flipped. We develop new closed form representations of sums of alternating harmonic numbers and reciprocal binomial coe cients. Then subtract Continuing in this way, we have found a way of rearranging the terms in the alternating harmonic series so that the sequence of partial sums for the rearranged series is unbounded and therefore diverges. The alternating harmonic series is defined as.